Kinematics of fluids
This free course explores probability and random processes, covering topics such as Bernoulli trials, Poisson processes, and multivariate Poisson processes. Learn to calculate probabilities and simulate events using relevant tables. Enhance your understanding of random processes and their applications.
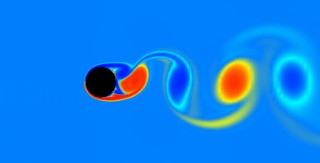
This free course examines the basic kinematics of two-dimensional fluid flows. Section 1 introduces the differential equations for pathlines and streamlines. Section 2 introduces a scalar field, called the stream function, which for an incompressible fluid provides an alternative method of modelling the flow and finding the streamlines. Sections 2 and 3 derive the stream functions for several simple two-dimensional flow types (the uniform flow, source, doublet and vortex), and suitable combinations of these are used to model more complicated flows. Section 4 introduces the idea of differentiation following the motion, and Euler’s equation is developed in Section 5.
Course learning outcomes
After studying this course, you should be able to:
Derive the pathlines and the streamlines in Cartesian and polar form from a velocity field
Derive the stream function from a velocity field
Model different flows from a combination of uniform flows, sources, sinks and doublets
Use the continuity equation to determine whether an inviscid flow is incompressible
Understand how Euler’s equation is derived, what it represents, and use it to find the pressure distribution from a velocity.






There are no reviews yet.